
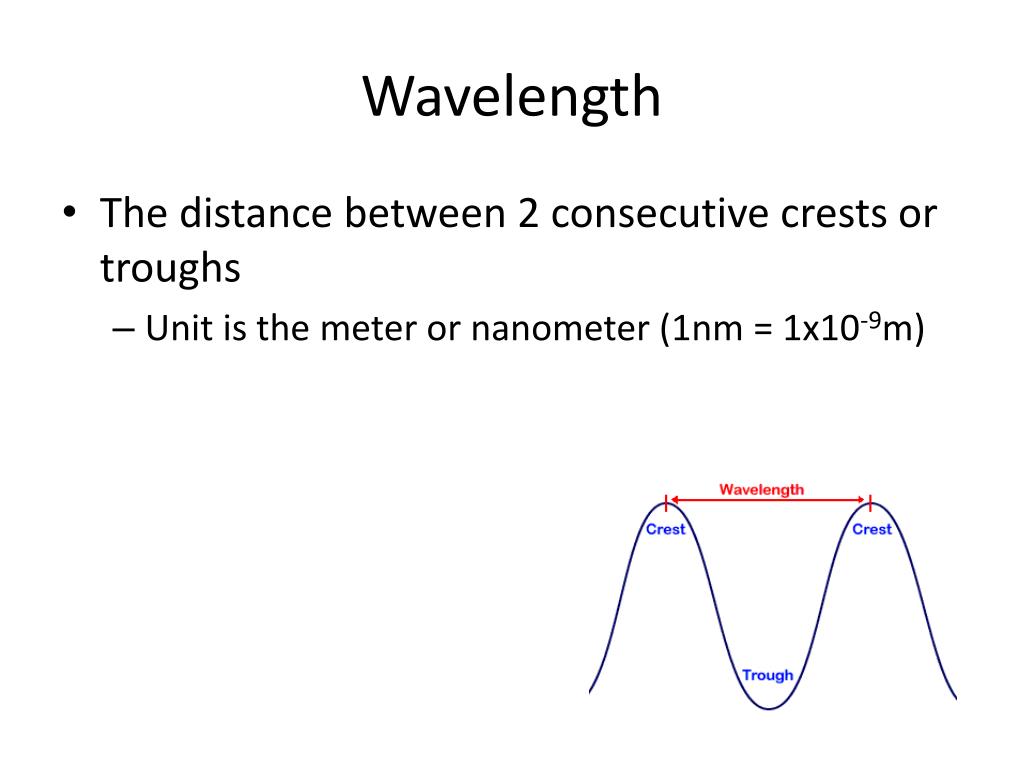
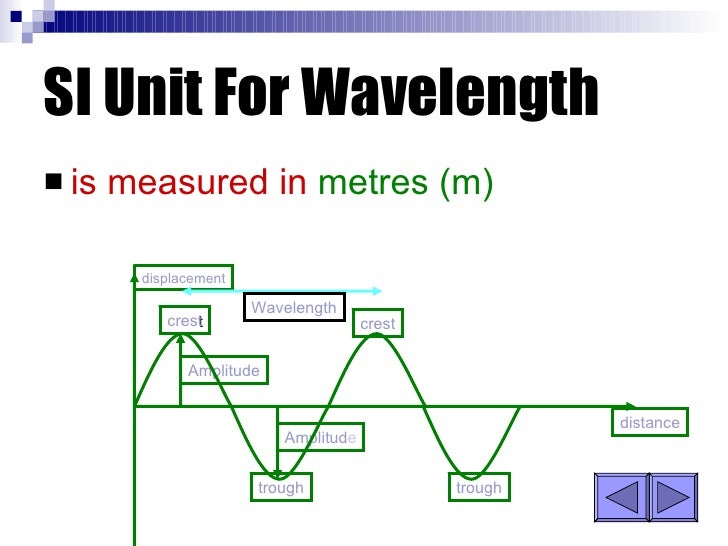
Doyne Farmer, in Philosophy of Information, 2008 6.1 Quantum states and the definition of a qubit Here, we introduce correction factors ε J, ε K and ε s in each unit realisation, i.e.,į. The quantum metrological triangle experiment is a consistency test to detect hidden errors that may exist in the realisation of the units. If a quantum current standard by SET is realised following the formula I = n S e f S, where n S is an integer, f S is the frequency of single-electron transfer, then these three quantum electrical standards form a so-called ‘quantum metrological triangle’ ( Fig. 5.19). In these effects, the voltage U and resistance (Hall resistance) R are quantised by the following formulas U = 1 K J n J f J and R = 1 n K R K, where K J = 2 e h is the Josephson constant, R K = h e 2 is the von-Klitzing constant, n J and n K are integers and f J is the frequency of the microwave driving the Josephson array. Since the values of Planck constant and elementary charge are fixed under the new SI system, the SI volt and ohm can be also realised independently by the Josephson effect and quantum Hall effect. The wavelength λ of a line in the hydrogen spectrum is related to the quantum numbers n and ℓ of the transitional orbits according to Bohr assumed that photons of energy |E f – E i| = hv = hc/λ are emitted or absorbed when electrons “jump” from an orbit with energy E i to one of energy E f. The total energy of an electron in the nth orbit is negative and proportional to 1/ n 2 the energy E n of an electron in the nth orbit is expressed in electron volts by E n = (−13.6/ n 2) eV. Similarly, the speed v n of the electron in the nth Bohr orbit is proportional to 1/ n. Using the coulombic force e 2/4π∈ o r 2 exerted on the electron by the photon as the centripetal force holding the electron in its circular orbit, it is found that the radius r n of the nth Bohr orbit is proportional to n 2, or r n = n 2 r 1, where r 1 is the radius of the first Bohr orbit. The Bohr theory postulates that the angular momentum mvr of the orbital electron is quantized: The angular momentum is restricted to values mvr = nh/ 2π, where n is the quantum number associated with the nth stationary orbit. All wavelengths of the hydrogen spectrum are accurately predicted by the Bohr theory, which assumes that the single electron in hydrogen is held in stable, nonradiating circular orbits by the coulombic attraction of the stationary central proton of the hydrogen atom. The spectral lines of hydrogen are records of the wavelengths of radiations emitted or absorbed by hydrogen atoms. Notice that the value of the stopping potential V o, measured in volts (V), is numerically equal to the maximum kinetic energy K max, measured in electron volts (eV). This photoelectric equation is sometimes written with K max expressed as eV o, where e is the electronic charge and V o is the stopping potential, the minimum potential difference (across the electrodes of a photoelectric cell) that will stop the most energetic photoelectrons. When a photon of energy hv > ϕ is incident upon a given metal surface, photoelectrons that have a maximum kinetic energy K max = hv – ϕ are emitted. The most energetic electrons inside a given metal are bound to that metal by an amount of energy called the work function ϕ of that particular metal.
This phenomenon is called the photoelectric effect. When light of sufficiently high frequency is incident upon a metal surface, photoelectrons are emitted from that surface. This momentum, or part of it, may be transferred to particles with which photons collide. Although a photon with wavelength λ has no mass, it possesses linear momentum of magnitude p = h/λ. A handy relationship between photon energy E in eV and radiation wavelength λ in nm frequently simplifies calculations in quantum problems: E(eV) = 1.24 × 10 3/λ(nm). Energies in quantum physics are commonly expressed in electron volts (1 eV = 1.6 × 10 −9 J) and wavelengths are typically given in nanometers (1 nm = 10 −9 m). The energy E of a photon is equal to hv = hc/λ, where v is the frequency of the electromagnetic radiation and λ is its wavelength. Planck's constant appears in association with photons, the particlelike aspect of electromagnetic waves it also appears in association with the de Broglie wavelength, the wavelike characteristic attributed to particles in quantum physics.Ī photon is a particlelike packet of electromagnetic energy. s, in a problem identifies that problem as being in the category of quantum physics.The presence of Planck's constant, h = 6.6 × 10 −34 J Tanner, in Physics for Students of Science and Engineering, 1985 23.6 Problem-Solving Summary


 0 kommentar(er)
0 kommentar(er)
